The Particular Solution of the Equation of Motion of a Single Particle under Lennard-Jones Potential
Keywords:
Lennard-Jones potential, Classical action, Euler-Lagrange equation, particular solution, simple harmonic oscillationAbstract
This research focuses on solving the particular solution of the equation of motion of a single particle under Leonard-Jones potential by defining the new parameter as x=(r/re )-6. We found that the Leonard Jones potential becomes parabolic in shape with the turning point shifted to r =1.122re. There are appear two conditions at r=0 and r=re. We applied this potential to the Euler-Lagrange equation, it was found that the equation was similar to the equation of motion of harmonic oscillation. However, when defining the boundary conditions for arbitrary constants, the arbitrary constants are complicated form, meanwhile, the equation of motion and their particular solutions we got are in a form that is suitable for further application in calculated the classical action of the system to determine the propagator and the wavefunction by path integral method.
References
J E. Jones, (1924). On the determination of molecular field- II. From the equation of state of a gas. Proc. R. Soc. Lond. A, 106(738), 463-477.
มฤเคนทร์ จอมเพชร, อาณาจักร พลจันทึก, ภ.พึ่งบุญ ปานศิลา, ณรงค์ฤทธิ์ มณีจิระปราการ และเสกสรร สุขะ-เสนา. (2562). อนุภาคในสถานะที่ถูกกักขังภายใต้ศักย์แบบเลนนาร์ด-โจนส์โดยอาศัยระเบียบวิธีเชิงตัวเลขแบบผลต่างจำกัด. J Sci Technol MSU, 38(4), 363-369.
นิคม ชูศิริ. (2541). อินทิกรัลตามเส้นทางของก๊าซอิเล็กตรอนในระบบสองมิติภายใต้อิทธิพลของแรงฮาร์มอนิกส์ สนามไฟฟ้า สนามแม่เหล็ก และศักย์แบบไร้ระเบียบ. มหาวิทยาลัยทักษิณ.
เอกพัน จันผง, อัฏฐชัย ถาวรสุวรรณ, วิทยา ทิพย์อักษร และ นิคม ชูศิริ. (2551). อินทิกรัลตามเส้นทางสำหรับกึ่งฮาร์มอนิกออสซิลเลเตอร. วารสารมหาวิทยาลัยทักษิณ. 11(2), 24-29.
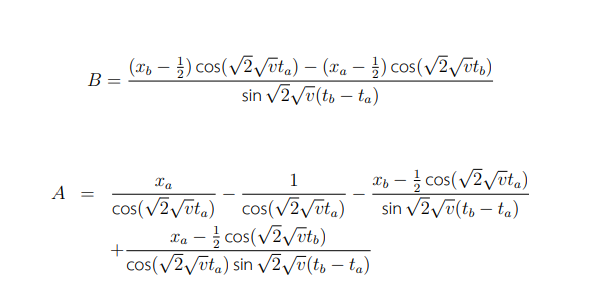
Downloads
Published
Issue
Section
License
บทความที่ได้รับการตีพิมพ์เป็นลิขสิทธิ์ของวารสาร มรภ.กพ. วิทยาศาสตร์ คณิตศาสตร์ และเทคโนโลยี
ข้อคิดเห็นใดๆ ที่ปรากฎในวารสารเป็นวรรณกรรมของผู้เขียนโดยเฉพาะ ซึ่งมหาวิทยาลัยราชภัฏกำแพงเพชรและบรรณาธิการไม่จำเป็นต้องเห็นด้วย


